In 1927 Heisenberg suggested the uncertainty principle, which can be formulated now as follows:
If one tries to describe the dynamical state of a quantum particle by methods of classical mechanics, then precision of such description is limited in principle. The classical state of the particle turns out to be badly defined.
In 2005 the certainty principle was suggested, which is formulated as follows:
If one describes the dynamical state of a quantum particle (system) by methods of quantum mechanics, then the quantum state of the particle (system) turns out to be well defined. This certainty of the quantum dynamical state means that “small” space-time transformations can not substantially change the quantum state.
Both principles are not just some misty philosophy about uncertainty and certainty, but they have quite rigorous mathematical formulations in the form of the following inequalities:
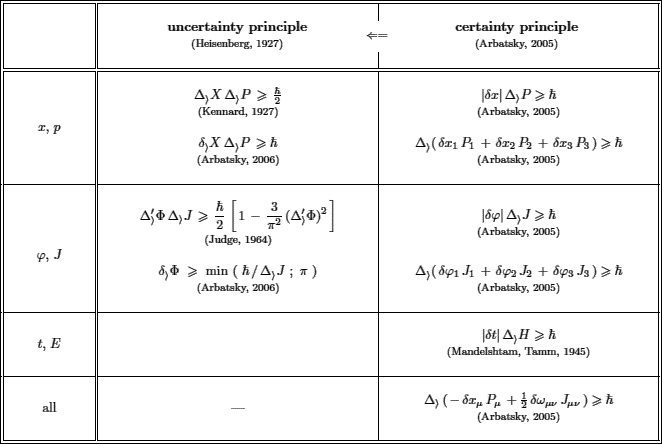
And the Heisenberg uncertainty principle is a consequence of the certainty principle. The certainty principle generalizes on the unified base both the uncertainty principle and the Mandelshtam-Tamm relation for energy and time (discovered in 1945).
A more detailed answer you can find in the paper The certainty principle (review).
An explanation for dummies is given in the article The certainty principle for dummies.
From the point of view of non-relativistic quantum mechanics, the certainty principle is just more general.
But from the point of view of relativistic quantum theory, it is more fundamental.
The matter is that for the theory of relativistic quantum systems the notion of “space coordinate”, as a quantum-mechanical observable (a self-adjoint operator), is not natural. Correspondingly, the uncertainty principle turns out to be sapless.
Because they did not know relativistic canonical quantization.
Fortunately, now that the certainty principle is already discovered, for its understanding it is sufficient a usual introductory course of quantum mechanics (knowledge of the RCQ-theory is not necessary).
A more concrete answer is given in the popular article The certainty principle for dummies.
 Home
Home