The elephant can not live in a dog kennel
The elephant can not be substantially moved in one step
April, 2006
(revised in August, 2006)
Abstract
Let us consider an elephant. The fact that the elephant is big, can be expressed in two ways:

The elephant can not live in a dog kennel

The elephant can not be substantially moved in one step
These two approaches can be developed in more detail:
If one tries to describe the position of the elephant by coordinates, it turns out to be not quite clear, position of which organ of the elephant should be measured: somebody, for example, more likes the trunk, and somebody more likes the tail. (A highly educated snob in this situation will make a say-so that in such a situation the position of the so called center of mass must be measured. But does the elephant really have such an organ?).
So, when one tries to describe the position of the elephant within the accuracy of the size of a dog, one encounters the uncertainty of its coordinate. And this uncertainty comes from the fact that the elephant is not a dog.
In the second approach, in order to characterize the size of the elephant one does not need to discriminate its separate organs and to look to their substantial space remoteness.
In contrast, one should place the elephant on a cart and move it till its new position is not overlapped with the initial. As a result of such an experiment, one will find that the necessary value of the displacement is large.
In this sense, one can say that the position of the elephant is well defined. And even if its position is defined with a substantial error, it will not be lost, anyway.
Possibly, a zoologist will think that the philosophy discussed here is just trivial. But he will not be right to the full extent.
The matter is that mathematics corresponding to these two approaches is different. And it is so different that for the microworld of quantum particles the second approach was realized 78 years later than the first one.
It is known that every quantum particle is a packet of the de Broglie waves. Under certain conditions, connected with the probability nature of the microworld, this wave packet can become very small and can behave like a classical particle. In this connection, people often talk about the wave-particle duality (though, at present, such a terminology seems to be a little archaic).
In the microworld the role of an elephant is played by the wave packet. In the simplest case it is described by some complex function of coordinates (i. e. for every point of space a complex number corresponds). This function is called a wave function. And it is supposed that the square of the absolute value of that function defines “the density of the probability to find the particle in a given place”. The wave function completely describes the dynamical state of the particle, i. e. it defines not only its state at the moment, but also allows to predict its state in the future.
The role of a dog in the microworld is played by a classical point particle. Its dynamical state, as you know, is defined by the coordinates and the velocity.
As a measure of how substantially the moved packet of de Broglie waves is differrent from the initial one the so called quantum angle is used, which is also known to mathematicians as a Fubini-Study metric (but here we will not refine such mathematical subtleties).
As a consequence of the two approaches described above, the space spread of the wave packet can be qualitatively described by two different principles: by the uncertainty principle and by the certainty principle.
The uncertainty principle can be formulated now as follows:
If one tries to describe the dynamical state of a quantum particle by methods of the classical mechanics, then the precision of such a description is limited in principle. The classical state of the particle turns out to be badly defined.
The certainty principle is formulated as follows:
If one describes the dynamical state of a quantum particle (system) by methods of the quantum mechanics, then the quantum state of the particle (system) turns out to be well defined. This certainty of the quantum dynamical state means that “small” space-time transformations can not substantially change the quantum state.
Both principles are not just some misty philosophy about uncertainty and certainty, but they have quite rigorous mathematical formulations in the form of the following inequalities:
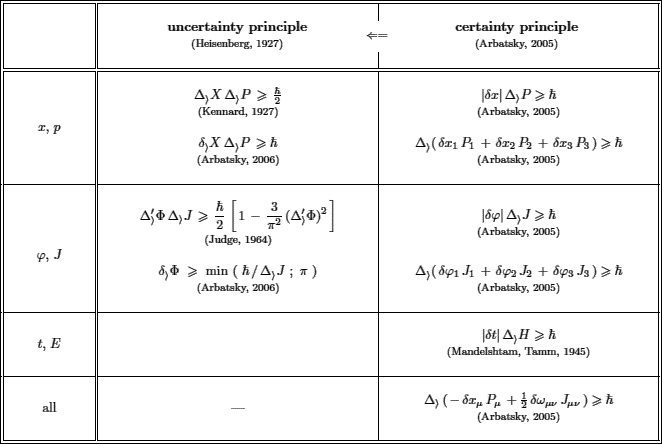
The detailed analysis shows that the uncertainty principle, from the mathematical point of view, is a rigorous consequence of the certainty principle. But not vice versa! So, the certainty principle is more general.
And what is more, from the point of view of the relativistic quantum theory, the certainty principle turns out to be more fundamental. This is because for relativistic quantum systems the notion of the coordinate is problematic.
In the theory of relativity space and time turn out to be very closely connected notions. And the absence of such an observable (in the sense of quantum mechanics) as a coordinate turns out to be closely connected with the absence of such an observable as time.
In contrast, in the non-relativistic quantum mechanics this connection can not be retraced. In this connection, an interesting historical misconception took place.
The greats of quantum mechanics for a long time searched for a formulation of an uncertainty relation for energy and time. General arguments, connected with the theory of relativity, pointed that such a relation seemingly should exist.
Then, in 1945 Mandelshtam and Tamm suggested a relation, which, as they thought, was the desired. So, in the table above they would put it in the left column, not in the right.
And there is nothing strange in that. Because the relativistic canonical quantization was not known at that time and nobody suspected the existence of the right column.
At present, we can say that the more fundamental exactly the right column is. And the existence of the left one is connected with specific features of the non-relativistic approximation.