The certainty principle
(review)

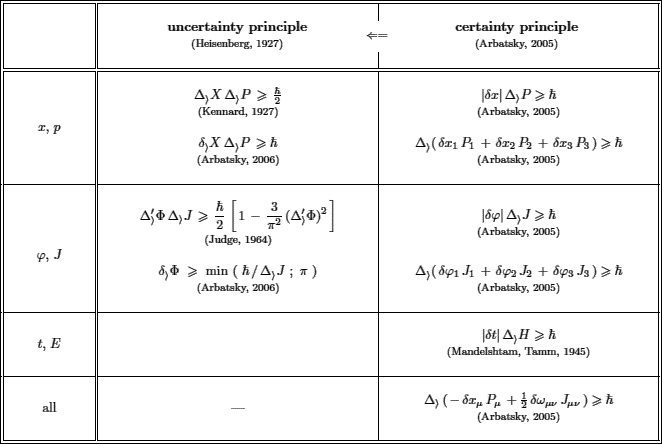
The certainty principle (2005) allowed to generalize and unify both the Heisenberg uncertainty principle (1927) and the Mandelshtam-Tamm relation (1945). It turned out to be applicable to any quantum systems, including relativistic quantum fields. One can even suppose, that it will finally turn out to be more fundamental than quantum mechanics and quantum field theory.

In the frame of the orthodox quantum theory the certainty principle can be considered as a mathematical theorem. Exactly this approach is assumed as a basis in this review. And with it the Heisenberg uncertainty principle and the Mandelshtam-Tamm relation are derived as consequences of the certainty principle. Another interesting consequence appears to be a simple uncertainty relation for angle and angular momentum, which, nevertheless, was not known before.

To wide extent, the certainty principle is not only a mathematical theorem, but also a gnoseological principle. This principle substantially complements the philosophy of quantum mechanics.